Cara lainnya dari pengerjaan soal No. 43

on Tidak ada komentar:

Jawaban No. 42. c dan 42.d

on Tidak ada komentar:

Jawaban untuk Soal 26. e

on Tidak ada komentar:

Eksponen dan Logaritma

A. Sifat-Sifat Eksponen dan Logaritma

\begin{array}{|l|l|}\hline \multicolumn{2}{|c|}{\textrm{Sifat yang berlaku pada}}\\\hline \textrm{Eksponens}&\textrm{Logaritma}\\\hline \displaystyle a^{n}=\underset{n\: \: faktor}{\underbrace{a\times a\times a\times \cdots \times a}}&^{a}\log b=c\: \Rightarrow \: a^{c}=b\\\hline \bullet \quad a^{p}\times a^{q}=a^{p+q}&\bullet \quad ^{a}\log x+\: ^{a}\log y=\: ^{a}\log xy\\\hline \bullet \quad a^{p}: a^{q}=a^{p-q}&\bullet \quad ^{a}\log x-\: ^{a}\log y=\: ^{a}\log \displaystyle \frac{x}{y}\\\hline \bullet \quad \left ( a^{p} \right )^{q}=a^{p.q}&\bullet \quad ^{a}\log x=\: \displaystyle \frac{^{m}\log x}{^{m}\log a}\\\hline \bullet \quad \displaystyle \sqrt[q]{a^{p}}=\displaystyle a^{ \left (\frac{p}{q} \right )}&\bullet \quad ^{a}\log b\: \times \: ^{b}\log c=\: ^{a}\log c\\\hline \bullet \quad \left ( a\times b \right )^{p}=a^{p}\: \times \: b^{p}&\bullet \quad ^{a^{m}}\log b^{n}=\displaystyle \frac{n}{m}\times \: ^{a}\log b\\\hline \bullet \quad \left ( \displaystyle \frac{a}{b} \right )^{p}=\displaystyle \frac{\displaystyle a^{p}}{\displaystyle b^{p}}&\bullet \quad \displaystyle a^{\: {^{a}}\log b}=b\\\hline \bullet \quad a^{-p}=\displaystyle \frac{1}{\displaystyle a^{p}}&\bullet \quad ^{a}\log b=\displaystyle \frac{1}{^{b}\log a}\\\hline \bullet \quad a^{0}=1,\: \: \: \: \: a\neq 0&\bullet \quad ^{a}\log 1=0\\\hline \bullet \quad a^{1}=1&\bullet \quad ^a\log a=1\\\hline \begin{cases} a,b\: \in \mathbb{R} \\ p,q\: \in \mathbb{Q} \end{cases}&\begin{cases} a\neq 0 & a>0\: \: (\textrm{bilangan pokok}) \\ x,y>0 & (\textrm{numerus}) \end{cases}\\\hline \end{array}

B. Persamaan Eksponen dan Logaritma

B.1 Persamaan Eksponen

\begin{array}{|l|l|l|}\hline \textrm{No}&\textrm{Bentuk}&\textrm{Syarat}\\\hline 1.&a^{f(x)}=1&a\neq 0,\quad \textrm{maka}\: \: f(x)=0\\\hline 2.&a^{f(x)}=a^{p}&a>0,\: \: a\neq 1,\quad \textrm{maka}\: \: f(x)=p\\\hline 3.&a^{f(x)}=a^{g(x)}&a>0,\: \: a\neq 1,\quad \textrm{maka}\: \: f(x)=g(x)\\\hline 4.&a^{f(x)}=b^{f(x)}&a\neq 0,\: b\neq 0\: ,\quad \textrm{maka}\: \: f(x)=0\\\hline 5.&f(x)^{g(x)}=1&\begin{cases} f(x)=1 & \\ g(x)=0, & \textrm{jika}\: \: f(x)\neq 0 \\ f(x)=-1, & \textrm{jika}\: \: g(x)=\: \textrm{genap} \end{cases}\\\hline 6.&f(x)^{g(x)}=f(x)^{h(x)}&\begin{cases} (i).\quad g(x)=h(x)& \\ (ii).\quad f(x)=1& \\ (iii).\quad f(x)=0,&g(x)>0,\: \: h(x)>0 \\ (iv).\quad f(x)=-1,&g(x)\: \textrm{dan}\: h(x)\: \: \\ &\textrm{keduanya ganjil atau genap} \end{cases}\\\hline 7.&g(x)^{f(x)}=h(x)^{f(x)}&\begin{cases} (i).\quad g(x) =h(x)& \\ (ii).\quad f(x)=0, & g(x)\neq 0,\: h(x)\neq 0 \end{cases}\\\hline 8.&A\left ( a^{f(x)} \right )^{2}+B\left ( a^{f(x)} \right )+C=0&a>0,\: \: a\neq 1\\\hline \end{array}

B.2 Persamaan Logaritma

\begin{array}{|l|l|l|}\hline \textrm{No}&\textrm{Bentuk}&\textrm{Syarat}\\\hline 1.&^a\log f(x)=0&f(x)>0,a>0,a\neq 0,\quad \textrm{maka}\: \: f(x)=1\\\hline 2.&^a\log f(x)=\: ^a\log p&a>0, a\neq 1, f(x)>0,p>0\quad \textrm{maka}\: \: f(x)=p\\\hline 3.&^a\log {f(x)}=\: ^a\log {g(x)}&a>0, a\neq 1,f(x)>,g(x)>0 \quad \textrm{maka}\: \: f(x)=g(x)\\\hline 4.&^a\log {f(x)}=\: ^b\log {f(x)}&a>0,b>0,a\neq 1, b\neq 1,f(x)>0,g(x)>0\\ && \textrm{maka}\: \: f(x)=0\\\hline 5.&^{h(x)}\log f(x)=\: ^{h(x)}\log g(x)&h(x)>0,h(x)\neq 1,f(x)>0,g(x)>0\\ && \textrm{maka}\: \: f(x)=g(x)\\\hline 6.&A(^a\log ^{2}f(x))+B(^a\log f(x))+C=0&\textrm{arahkan ke persamaan kuadrat}\\\hline 7.&a^{f(x)}=b^{g(x)}&\textrm{gunakan aturan logaritma}\\\hline \end{array}

C. Pertidaksamaan Eksponen dan Logaritma

C. 1 Pertidaksamaan Eksponen

\begin{array}{|l|l|}\hline a>1&0<a<1\\\hline a^{f(x)}\leq a^{g(x)}\Rightarrow f(x)\leq g(x)&a^{f(x)}\leq a^{g(x)}\Rightarrow f(x)\geq g(x)\\\hline a^{f(x)}< a^{g(x)}\Rightarrow f(x)< g(x)&a^{f(x)}< a^{g(x)}\Rightarrow f(x)> g(x)\\\hline a^{f(x)}\geq a^{g(x)}\Rightarrow f(x)\geq g(x)&a^{f(x)}\geq a^{g(x)}\Rightarrow f(x)\leq g(x)\\\hline a^{f(x)}> a^{g(x)}\Rightarrow f(x)> g(x)&a^{f(x)}> a^{g(x)}\Rightarrow f(x)< g(x)\\\hline \end{array}

C. 2 Pertidaksamaan Logaritma 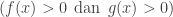 \left (f(x)>0\: \: \textrm{dan}\: \: g(x)>0 \right )

\begin{array}{|l|l|}\hline a>1&0<a<1\\\hline ^a\log f(x)\leq \: ^a\log g(x)\Rightarrow f(x)\leq g(x)&^a\log f(x)\leq \: ^a\log g(x)\Rightarrow f(x)\geq g(x)\\\hline ^a\log f(x)< \: ^a\log g(x)\Rightarrow f(x)< g(x)&^a\log f(x)< \: ^a\log g(x)\Rightarrow f(x)> g(x)\\\hline ^a\log f(x)\geq \: ^a\log g(x)\Rightarrow f(x)\geq g(x)&^a\log f(x)\geq \: ^a\log g(x)\Rightarrow f(x)\leq g(x)\\\hline ^a\log f(x)> \: ^a\log g(x)\Rightarrow f(x)> g(x)&^a\log f(x)> \: ^a\log g(x)\Rightarrow f(x)< g(x)\\\hline \end{array}.

D. Grafik Fungsi Eksponen dan Logaritma

 D.1 Grafik fungsi Eksponen


D.2 Grafik fungsi Logaritma

\LARGE{\fbox{\LARGE{\fbox{CONTOH SOAL}}}}.

\begin{array}{ll}\\ \fbox{1}.&\textrm{Tentukanlah nilai dari bilangan-bilangan berikut ini}! \end{array}\\ \begin{array}{llllllll}\\ .\quad\quad &a.&27^{\frac{1}{3}}&k.&\left ( \displaystyle \frac{2^{3}.3^{-2}}{2^{-5}.3} \right )^{\displaystyle \frac{1}{2}}&u.&\displaystyle \frac{\left ( a^{2}.b^{-1} \right )^{\frac{1}{2}}\sqrt{a^{6}.b^{\frac{5}{3}}.c^{-2}}}{\left ( a^{3}.b^{-5}.c^{-3} \right )^{\frac{1}{3}}}\\ &b.&32^{^{\frac{2}{5}}}&l.&\displaystyle \frac{\sqrt{2}.\sqrt[3]{8}}{(2)^{\frac{1}{3}}.\sqrt[4]{16^{2}}}&v.&\displaystyle \frac{x^{2}.y^{7}}{x^{3}.y^{5}}\\ &c.&\left ( \displaystyle \frac{9}{16} \right )^{\displaystyle \frac{3}{2}}&m.&\left ( \displaystyle \frac{\sqrt{2}.2\sqrt{6}}{\sqrt{3}.\sqrt[3]{9}} \right )^{\displaystyle \frac{1}{2}}&w.&\left ( \displaystyle \frac{2x^{3}}{y^{2}}:\frac{4x^{6}}{4y^{5}} \right ).\displaystyle \frac{3x^{2}-2y}{3y}\\ &d.&\left ( \displaystyle \frac{1}{125} \right )^{-\frac{1}{3}}&n.&\displaystyle \frac{2.3^{-\frac{1}{2}}-2+3.2^{-\frac{1}{2}}}{2.3^{-\frac{1}{2}}-3.2^{-\frac{1}{2}}}&x.&\left (\sqrt[3]{x^{2}.yz^{3}} \right ).x^{-1}.y^{-2}\\ &e.&\left ( \displaystyle \frac{2}{3} \right )^{\displaystyle \frac{2}{3}}.\left ( \displaystyle \frac{3}{2} \right )^{-\displaystyle \frac{1}{3}}&o.&-3\sqrt{6}+4\sqrt{3}-2\sqrt{81}&y.&\displaystyle \frac{\sqrt{x}.\sqrt{x^{2}y^{3}}.\sqrt{xy^{2}}}{\sqrt[4]{x}.\sqrt[3]{y}}\\ &f.&\left ( \displaystyle \frac{1}{5^{3}} \right )^{-1}.\left ( \displaystyle \frac{1}{5^{2}} \right )^{2}&p.&\sqrt{250}-\sqrt{50}+15\sqrt{2}&z.&\displaystyle \frac{\left ( x^{2} \right )^{3}}{x^{4}}:\left ( \frac{x^{3}}{\left ( x^{3} \right )^{2}} \right )^{-2} \end{array}
\begin{array}{llllllll}\\ .\quad\quad&g.&\displaystyle \frac{\sqrt{3}.\sqrt{15}}{\sqrt{5}}\quad\qquad \: \: &q.&\sqrt[2]{75}-\sqrt[4]{27}+\sqrt[3]{128}\\ &h.&\displaystyle \frac{2\sqrt{3}.\sqrt{24}}{\sqrt{7}.3\sqrt{14}}&r.&\displaystyle \frac{5\sqrt{5}+2\sqrt{5}}{5-3\sqrt{5}}\\ &i&\displaystyle \frac{\sqrt{5}.\sqrt[2]{2^{3}}}{2.\sqrt[3]{3}}&s.&\sqrt{2\sqrt{2\sqrt{2\sqrt{2\sqrt{2\sqrt{\cdots }}}}}}\\ &j.&\displaystyle \frac{\sqrt{3}.\sqrt[3]{2}}{\left ( \frac{4}{9} \right )^{3}.\left ( \frac{2}{3} \right )^{-2}}&t.&\left ( 4^{\frac{1}{2}} \right )^{\frac{1}{2}}\left ( 2^{-2} \right )^{-2}.\sqrt[3]{0,125}.\left ( 0,25 \right ).\displaystyle \frac{1}{2}\sqrt{\frac{1}{2}} \end{array}

Pembahasan:

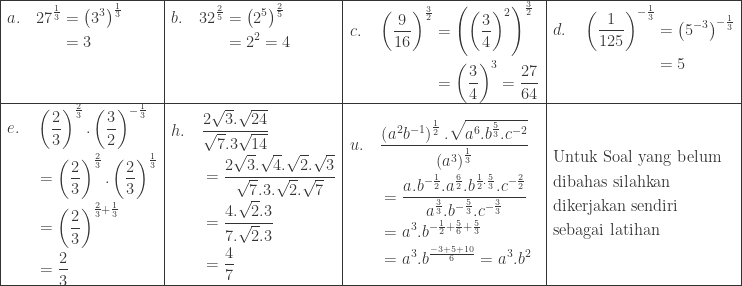
\begin{array}{|l|l|l|l|}\hline \begin{aligned}a.\quad 27^{\frac{1}{3}}&=\left ( 3^{3} \right )^{\frac{1}{3}}\\ &=3\\ &\\ & \end{aligned}&\begin{aligned}b.\quad 32^{\frac{2}{5}}&=\left ( 2^{5} \right )^{\frac{2}{5}}\\ &=2^{2}=4\\ &\\ & \end{aligned}&\begin{aligned}c.\quad \left ( \displaystyle \frac{9}{16} \right )^{\frac{3}{2}}&=\left (\left ( \displaystyle \frac{3}{4} \right )^{2} \right )^{\frac{3}{2}}\\ &=\left ( \displaystyle \frac{3}{4} \right )^{3}=\frac{27}{64} \end{aligned}&\begin{aligned}d.\quad \left ( \displaystyle \frac{1}{125} \right )^{-\frac{1}{3}}&=\left ( 5^{-3} \right )^{-\frac{1}{3}}\\ &=5\\ & \end{aligned}\\\hline \begin{aligned}e.\quad &\left ( \frac{2}{3} \right )^{\frac{2}{3}}.\left ( \frac{3}{2} \right )^{-\frac{1}{3}}\\ &=\left ( \frac{2}{3} \right )^{\frac{2}{3}}.\left ( \frac{2}{3} \right )^{\frac{1}{3}}\\ &=\left ( \frac{2}{3} \right )^{\frac{2}{3}+\frac{1}{3}}\\ &=\frac{2}{3} \end{aligned}&\begin{aligned}h.\quad &\displaystyle \frac{2\sqrt{3}.\sqrt{24}}{\sqrt{7}.3\sqrt{14}}\\ &=\displaystyle \frac{2\sqrt{3}.\sqrt{4}.\sqrt{2}.\sqrt{3}}{\sqrt{7}.3.\sqrt{2}.\sqrt{7}}\\ &=\frac{4.\sqrt{2}.3}{7.\sqrt{2}.3}\\ &=\frac{4}{7} \end{aligned}&\begin{aligned}u.\quad &\displaystyle \frac{\left ( a^{2}b^{-1} \right )^{\frac{1}{2}}.\sqrt{a^{6}.b^{\frac{5}{3}}.c^{-2}}}{\left ( a^{3} \right )^{\frac{1}{3}}}\\ &=\displaystyle \frac{a.b^{-\frac{1}{2}}.a^{\frac{6}{2}}.b^{\frac{1}{2}.\frac{5}{3}}.c^{-\frac{2}{2}}}{a^{\frac{3}{3}}.b^{-\frac{5}{3}}.c^{-\frac{3}{3}}}\\ &=a^{3}.b^{-\frac{1}{2}+\frac{5}{6}+\frac{5}{3}}\\ &=a^{3}.b^{\frac{-3+5+10}{6}}=a^{3}.b^{2} \end{aligned}&\begin{aligned}&\textrm{Untuk Soal yang belum}\\ &\textrm{dibahas silahkan}\\ &\textrm{dikerjakan sendiri}\\ &\textrm{sebagai latihan} \end{aligned}\\\hline \end{array}

\begin{array}{ll}\\ \fbox{2}.&\textrm{Tentukanlah himpunan penyelesaian (HP) dari}\\ &\textrm{a}.\quad 3^{2x-1}=1\\ &\textrm{b}.\quad 4^{x^{2}+3x-10}=1\\ &\textrm{c}.\quad 5^{3x^{2}+2x-1}=1\\ &\textrm{d}.\quad (9)^{2x+\frac{1}{2}}.\left ( \displaystyle \frac{1}{27} \right )^{3x^{2}+2}=1\\ &\textrm{e}.\quad \left ( \displaystyle \frac{1}{2} \right )^{2x-3}.(8)^{3x+1}=1\\ &\textrm{f}.\quad \displaystyle \frac{\sqrt[3]{\left ( 0,0008 \right )^{7-2x}}}{\left ( 0,2 \right )^{-4x+5}}=1\: \: ...(\textbf{SPMB 2005})\end{array}

Pembahasan:

\begin{array}{|l|l|l|}\hline \begin{aligned}a.\quad 3^{2x-1}&=1\\ 3^{2x-1}&=3^{0}\\ 2x-1&=0\\ 2x&=1\\ x&=\displaystyle \frac{1}{2}\\ \textrm{HP}=&\left \{ \displaystyle \frac{1}{2} \right \}\\ &\\ &\\ & \end{aligned}&\begin{aligned}e.\quad \left ( \displaystyle \frac{1}{2} \right )^{2x-3}.(8)^{3x+1}&=1\\ (2^{3-2x}).(2^{3})^{3x+1}&=2^{0}\\ 2^{3-2x+9x+3}&=2^{0}\\ 7x+6&=0\\ 7x&=-6\\ x&=-\displaystyle \frac{6}{7}\\ \textrm{HP}=\left \{ -\displaystyle \frac{6}{7} \right \}&\\ & \end{aligned}&\begin{aligned}f.\quad \displaystyle \frac{\sqrt[3]{\left ( 0,0008 \right )^{7-2x}}}{\left ( 0,2 \right )^{-4x+5}}&=1\: \: ...(\textbf{SPMB 2005})\\ \displaystyle \frac{((0,2)^{3})^{\frac{7-2x}{3}}}{(0,2)^{-4x+5}}&=(0,2)^{0}\\ (0,2)^{7-2x-(-4x+5)}&=(0,2)^{0}\\ 7-2x+4x-5&=0\\ 2x+2&=0\\ 2&=-2\\ x&=-1\\ \textrm{HP}=\left \{ -1 \right \}& \end{aligned}\\\hline \end{array}

\begin{array}{ll}\\ \fbox{3}.&\textrm{Tentukanlah himpunan penyelesaian (HP) dari}\\ &\textrm{a}.\quad 3^{2x-5}=3^{5}\\ &\textrm{b}.\quad 2^{2x+3}.\left (\displaystyle \frac{1}{8} \right )^{x-3}=\displaystyle \frac{1}{64}\\ &\textrm{c}.\quad (2)^{5x^{2}-3x}.\left ( \displaystyle \frac{1}{32} \right )^{5}=32^{5}\\ &\textrm{d}.\quad \left ( \displaystyle \frac{1}{9} \right )^{-x^{2}}.\left ( 3^{2} \right )^{3x-3}=\displaystyle 9^{3^{2}}\\ &\textrm{e}.\quad (4)^{-2x^{2}+3x}.\left ( \displaystyle \frac{1}{4} \right )^{3}=2^{-2}.\left ( \displaystyle \frac{1}{8} \right )^{-2}\\ &\textrm{f}.\quad \displaystyle \frac{27}{3^{2x-1}}=81^{-0,125}\: \: ...(\textbf{SPMB 2004})\end{array}
Pembahasan:
\begin{array}{|l|l|}\hline \begin{aligned}a.\quad 3^{2x-5}&=3^{5}\\ a^{f(x)}&=a^{p}\\ f(x)&=p\\ 2x-5&=5\\ 2x&=10\\ x&=5\\ \textrm{HP}=&\left \{ 5 \right \}\\ &\\ &\\ &\\ &\\ &\end{aligned}&\begin{aligned}f.\quad \displaystyle \frac{27}{3^{2x-1}}&=81^{-0,125}\cdots (\textbf{SPMB 2004})\\ \displaystyle \frac{3^{3}}{3^{2x-1}}&=(3^{4})^{-\frac{1}{8}}\\ 3^{3-(2x-1)}&=3^{-\frac{4}{8}}\\ 3-(2x-1)&=-\frac{4}{8}\\ 4-2x&=-\frac{1}{2}\\ 8-4x&=-1\\ -4x&=-1-8\\ x&=\frac{9}{4}\\ \textrm{HP}=&\left \{ \frac{9}{4} \right \}\end{aligned}\\\hline \end{array}
\begin{array}{ll}\\ \fbox{4}.&\textrm{Tentukanlah himpunan penyelesaian (HP) dari}\\ &\textrm{a}.\quad \sqrt{3^{2x+1}}=9^{x-2}\\ &\textrm{b}.\quad \left ( \displaystyle \frac{1}{3} \right )^{2x-3}.3^{x+5}=\left ( \displaystyle \frac{1}{27} \right )^{2x-10}\\ &\textrm{c}.\quad (125)^{(x^{2}-3x-4)}=(5)^{(x^{2}-2x-3)}\\ &\textrm{d}.\quad (2^{2})^{\displaystyle \sqrt{x^{3}+2x^{2}-3x-6}}=\left ( \displaystyle \frac{1}{2} \right )^{-\displaystyle \sqrt{4x^{2}+4x-8}}\\ &\textrm{e}.\quad (4)^{(x^{2}+2x-1)}.\left ( \displaystyle \frac{1}{8} \right )^{3x-4}=\left ( \displaystyle \frac{1}{2} \right )^{2x}\\ &\textrm{f}.\quad (\sqrt{2})^{4x^{2}-8x+12}.\left ( \sqrt[3]{8} \right )^{3x+5}=\left ( \displaystyle \frac{1}{4} \right )^{4x-3}.(2)^{6x+5}\\ &\textrm{g}.\quad 9^{x^{2}-3x+1}+9^{-3x+x^{2}}=20-10\left ( 3^{x^{2}-3x} \right )\: \: ...(\textbf{SPMB 2004})\end{array}
Pembahasan:
\begin{array}{|l|l|l|}\hline \begin{aligned}a.\quad \sqrt{3^{2x+1}}&=9^{x-2}\\ a^{f(x)}&=a^{g(x)}\\ f(x)&=g(x)\\ \displaystyle (3)^{\frac{2x+1}{2}}&=(3^{2})^{x-2}\\ \displaystyle \frac{2x+1}{2}&=2(x-2)\\ 2x+1&=4x-8\\ -2x&=-9\\ x&=\displaystyle \frac{9}{2}\\ \textrm{HP}=&\left \{ \frac{9}{2} \right \} \end{aligned}&\begin{aligned}b.\quad \left ( \displaystyle \frac{1}{3} \right )^{2x-3}.3^{x+5}&=\left ( \displaystyle \frac{1}{27} \right )^{2x-10}\\ (3^{-1})^{(2x-3)}.3^{x+5}&=(3^{-3})^{(2x-10)}\\ 3^{(-2x+3)+(x+5)}&=3^{10-2x}\\ -2x+3+x+5&=10-2x\\ x&=10-8\\ x&=2\\ \textrm{HP}=\left \{ 2 \right \}&\\ &\\ &\\ & \end{aligned}&\begin{aligned}c.\quad (125)^{x^{2}-3x-4}&=(5)^{x^{2}-2x-3}\\ (5^{3})^{x^{2}-3x-4}&=(5)^{x^{2}-2x-3}\\ 3x^{2}-9x-12&=x^{2}-2x-3\\ 2x^{2}-7x-9&=0\\ (x+1)(2x-9)&=0\\ x=-1\: \: \textrm{V}\: \: x=\frac{9}{2}\\ \textrm{HP}=&\left \{ -1,\frac{9}{2} \right \}\\ &\\ &\\ & \end{aligned}\\\hline \multicolumn{3}{|l|}{\begin{aligned}g.\qquad\quad\quad\quad 9^{x^{2}-3x+1}+9^{-3x+x^{2}}&=20-10\left ( 3^{x^{2}-3x} \right )\\ \textrm{misalkan}\: \: 3^{x^{2}-3x}&=p\\ 9^{x^{2}-3x}.9+9^{x^{2}-3x}&=20-10\left ( 3^{x^{2}-3x} \right )\\ 9.\left ( 3^{2.(x^{2}-3x)} \right )+\left ( 3^{2.(x^{2}-3x)} \right )&=20-10\left ( 3^{x^{2}-3x} \right )\\ 10.\left ( 3^{x^{2}-3x} \right )^{2}&=20-10\left ( 3^{x^{2}-3x} \right )\\ 10p^{2}&=20-10p\\ p^{2}&=2-p\\ p^{2}+p-2&=0\\ (p+2)(p-1)&=0\\ p=-2\: (\textrm{tdk mungkin})\: \: \textrm{V}\: \: p=1&\\ \textrm{sehingga}\quad p=3^{x^{2}-3x}&=1\\ 3^{x^{2}-3x}&=3^{0}\\ x^{2}-3x&=0\\ x(x-3)&=0\\ x=1\: \: \textrm{V}\: \: x&=3\\ \textrm{HP}=&\left \{ 1,3 \right \} \end{aligned}}\\\hline \end{array}
Sumber Referensi

  1. Kuntarti, Sulistiyono dan Sri Kurnianingsih. 2005. Matematika untuk SMA dan MA Kelas XII Program Ilmu Alam. Jakarta: Gelora Aksara Pratama.
  2. Kuntarti, Sulistiyono dan Sri Kurnianingsih. 2007. Matematika  SMA dan MA untuk Kelas XII Semester 2 Program IPA Standar Isi 2006. Jakarta: esis.
  3. Soetiyono, Kamta Agus Sajaka, Sigit suprijanto, Marwanta, Suwarsini Murniati, dan Herynugroho. 2007. Matematika Interaktif 3B Sekolah Menengah Atas Kelas XII Program Ilmu Pengetahuan Alam. Jakarta: Yudistira.
  4. Tim IGMP Matematika SMA. ….. . Tabloid Matematika Kurikulum 2006. Semarang: CV. Sarana Ilmu.
  5. Tung, Khoe Yao. 2012. Pintar Matematika SMA Kelas XII IPA Untuk Olimpiade dan Pengayaan Pelajaran. Yogyakarta: ANDI.

on Tidak ada komentar:
Berikut untuk jawaban 11.e



dan untuk jawaban 11.f  adalah




on Tidak ada komentar:
Langganan: Postingan (Atom)